Introduction
There is a limit to how near an orbiting object can get to a black hole without becoming "lost in space." There is a limit to the radius reduction of a condensing or contracting mass, before it must change state. The limit is the number three. Is the limiting radius for spherical phenemonae a one-digit dimensionless whole number?
The answer to this, and many other questions, lies in revolutionary discoveries by Howard Lawrence Schriefer in pursuit of a Unified Theory of the universe.

Hysterises analysis based upon the Schriefer Unified Theory computationally exhibits that there is a limit to reduction of a spherical mass radius for contraction of that sphere without the necessity of a phase change. In other words, the limit of volume for a sphere to contract, without having to have a phase change, is where the radius is dimensionlessly equal to three. If the radius is less than three, contraction cannot occur without a change in compositional structure.
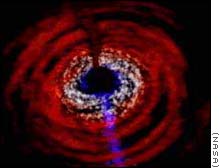
Black hole effects are reported to be strongest near the Schwarzchild Radius, Rs = 2GM/c2. A black hole is defined as an object whose mass lies entirely within its Schwarzchild Radius. The last stable orbit at 3Rs is the closest at which a material particle can orbit the hole; any closer, and the particle must move radially inward or outward. Radially inward results in irrevocable entry into the black hole. A good site to visit for clear discussion of black holes is Chapter 9, at astro.virginia.edu by the University of Virginia, Department of Astronomy.

